Shortcomings of the IRR
Now here is another example. Project B and Project B again you get $100 million today, you expect to put down $300 million a year from now, and you expect to get $250 million a year from now. And we are still dealing; let us say with the same company that has the same discount rate of 12%. Now, let us look at the picture first and, and remember.
By definition, the internal rate of return is the rate, the discount rate for which the NPV is equal to zero.
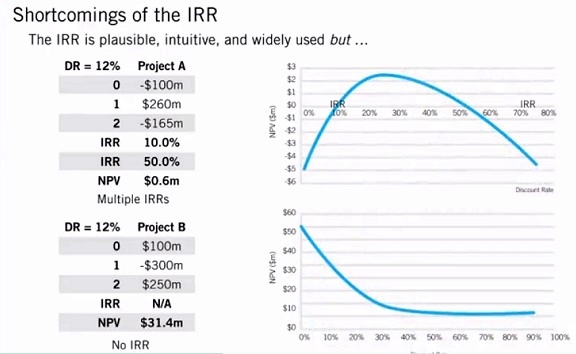
But look at that picture there. That blue line never crosses the horizontal line and that means that there’s no solution for that. In fact, if you throw these three cash flows into Excel and you apply the IRR command, that then you are going to get no solution. That is Excel’s way of saying, I cannot solve for this. And the reason why you cannot solve for this, you have it in front of your eyes. That blue line never crosses the horizontal axis. There is no discount rate for which this NPV is equal to zero and obviously, while in this case, we cannot use the IRR, if we cannot compute a number, we have nothing to compare to, to the discount rate, and therefore we cannot use the IRR.
Now, again, we fall back to our rule that we said before, we calculate the NPV, we got over $31 million of positive NPV and therefore we should go ahead with this project. But, the problem to highlight here is that it may well be the case that you are trying to find the IRR of your project and this project does not have. You cannot calculate an IRR. Therefore, we have two opposite problems.
- We can have no IRR
Or
- We can have more than one IRR.
Both cases are problematic. In both cases, you need to fall back on whatever the NPV happens to recommend. Now, there are many other problems that we could actually discuss, and in fact, the complementary reading that goes with this session it will explore more than the problems that we are discussing here in our limited time. However, there is one more that I want to highlight because it is important and actually, it is a problem that you may encounter in practice more than once. Before we get to that problem, let me ask you that simple question. Suppose that I give you this option. Option number one, you give me $1 today and I give you back $2 next week. Now let us suppose you believe me that I am going to give you those two dollars next week so let’s consider two propositions that I will put before you as risk-free propositions.
So proposition number one, give me $1 today, I give you back $2 a week from now. Proposition number two, you give me a million dollars today and I give you back $1.9 million next week. Moreover, I know that you may think that I may not give you back $1.9 million next week but again bear with me. Trust me. I am going to give you those $1.9 million. This is a risk-free proposition for you and I the only thing I say is to look either you have to go for option one or you have to go for option two. Which one would you choose? Well here is the thing with that. If you think exclusively in terms of return. Option number one. Gives you 100% return. You give me one $1 today; I will give you $2 next week. Your return between one week and the next is exactly 100%. Now if you give me $1 million today, and I give you back $1.9 million next week, your return is not 100%. It is only 90%. That is a good return for one week. Now but still, you know, what really counts here is that in one week you are going to be $900,000 richer.
So would you prefer to be $1 richer in a week or $900,000 richer in one week?
Well although the return on the second project is lower. You know, every time that I ask this question to my students, everybody chooses option two. You obviously prefer to go from. One million dollars to $1.9 million rather than from $1 to $2 although the return on the first proposition is higher than the return on the second proposition.
Why is this example important?
Well, we are going to see another example now in just a minute to compliment this, but notice that what this example is telling you is that return only is not the only thing that matters. That is, in the first case you get 100%, in the second case, you get 90%, but you’re also thinking in terms of the amount of money that you put in your pocket. That is important, why? Well, let us look at these two possibilities.
We have two very simple projects. Project C, we put $100 million today. We expect to get $150 million a year from now. Project D, and we have to go either/or. Either we go for one, or we will go for the other. Project D, I need to put $200 million today, and I get $280 million a year from now. We are still dealing with a company that has a discount rate of 12% and the question is you know what we call before competitive projects. Should we go for Project C or should we go for Project D? Well, there is more than one way of looking at that. First, let us look at the internal rates of return. The IRR of Project C is 50%. The IRR of Project D is 40%. So if we go by the, I wouldn’t call it informal, but the rule we suggested before, that the higher the IRR the better the project, then we should go for Project C. But, remember, we also said, but be careful with this rule, because it is not universally true.
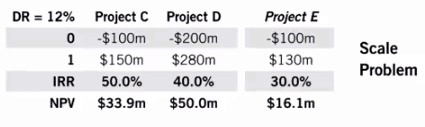
There are some loopholes, and we will talk about it. Well, we are talking about it right now. It looks like if we go by IRR, the first project, C has 50% IRR. The second project, D has 40% IRR. We should go for Project C. However, look at the NPVs. The NPVs tell you exactly the opposite thing. That it is the Project C has an NPV of almost 34 million, but Project D has an NPV of 50 million, and so this is one situation in which the NPV rule and the IRR rule actually pulling in different directions. And as we said before, whenever you have one rule suggesting that you should do one thing, and another rule, the NPV rule, suggesting the opposite, you should always fall back on the NPV rule. Therefore, in this particular case, although the IRR of Project D is lower than the IRR of Project C, because the NPV of Project D is higher than that of Project C you should actually choose Project D. Now there is another way of seeing why we need to go to Project D. Moreover, let me just put that, those cash flows. And notice that we are calling that Project E. And we are calling project in the quotation because it is not really a project. If, if you actually pay attention to those numbers that actually is the differential cash flow between Project D and Project C. In other words, if I invest in Project D as opposed to Project C, I need to put an additional $100 million, which is a number that you have right there, and if I invest in Project D as opposed to Project C, I expect to get an additional $130 million, so instead of getting 150 million, I’m going to get 280. Instead of putting down a hundred million, we’re going to get 200.
Therefore, as you see if you subtract the two cash flows in, in the column Project D to the two cash flows in the column Project D, then you are going to get the cash flows in the project E. Now the question becomes, does it pay to put an additional $100 million in Project D in order to expect an additional $130 million, in, in Project E. And if you actually calculate the IRR of that. It is clearly positive at 30%, which is higher than our discount rate of 12%. It has a clearly positive net present value and that says yes it does pay to put an additional $100 million in Project D in order to expect an additional $130 million in Project D. So one way or the other what we’re coming up to the same solution we, we come up that we should invest in Project D by comparing the NPVs or by looking at the differential cash flows and the internal rate of return on the NPV of those differential cash flows. This is what is called the scale problem.